I just happened across this on YouTube today. I don’t recall ever hearing about this “paradox” before, but apparently it has sparked some heated debate over the years.
The Dome Paradox: A Loophole in Newton’s Laws
John Norton’s 2003 paper: (Microsoft Word - 003004.doc)
Here is the main part of his argument: The Dome
This is one of David’s recent papers that he shared with me. The finitist approach to physics has increasing adherants.
It’s remarkable that this individual is professor at a reputable university. Aside from the many unimportant errors in this paper, there is a fundamental misunderstanding of calculus. Prof. Norton integrates the equation of motion for this particle without accounting for a constant of integration: velocity is the integral of acceleration with an undetermined constant. That constant is also known as an initial condition in physics. He specifies one initial condition, r(0)=0 but implicitly makes the initial velocity nonzero, viz. r(t) = (1/144) (t-T)^4, which has a discontinuous derivative at t=T. Well, surprise, surprise: if the initial velocity is not zero, the particle will move!
tl;dr
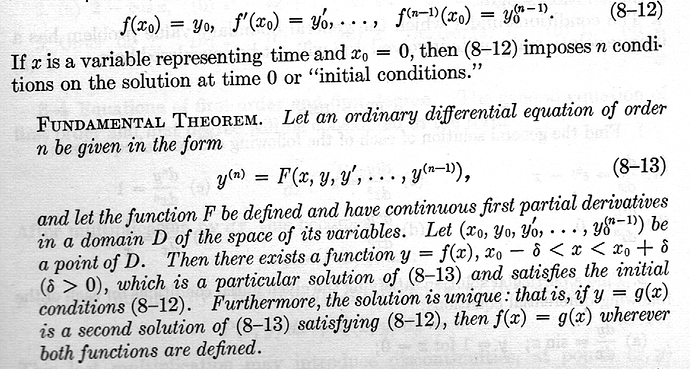
Newton’s equation of motion requires initial conditions of position and velocity because it is a second-order differential equation.
My real motive for posting this comment is that it gives me the opportunity to tell my favorite calculus joke:
Two mathematics professors are having a pint or two at the pub.
Prof. A: Most people don’t know anything about calculus. I bet our waitress doesn’t what the derivative of e^x is.
Prof. B: I wouldn’t be so sure about that.
A: We’ll see.
[Prof. A goes off to the men’s room.]
B: [to waitress] I want to play a prank on my colleague. When he gets back, he’s going to ask you some questions. Just answer “e to the x.”
[Prof. B returns]
A: [to waitress] What is the derivative of e^x?
Waitress: e^x
A: What’s the integral of e^x?
Waitress: e^x… plus an arbitrary constant.
The waitress in the joke has a better command of calculus than Prof. Norton.
Correction: My claim that r’(0) (initial velocity) is nnonzero is wrong! However, the rest of the argument holds up. By changing the function from r(t)=0 to r(t)=(1/144) (t-T)^4, Prof. Norton has introduced a discontinuity that, unsurprisingly, just happens to be where the velocity starts to be nonzero. In short, he’s rigged it so the mass starts to move at t=T.
There are two fundamental problems with the problem as formulated:
The first of these problems is easy to fix by using cartesian coordinates. The setup can be simplified even further by making it one-dimensional motion: a bead moving frictionlessly on a wire of the same shape as a cross-section of the dome. Not realizable in practice but that’s okay.
The second problem is trickier. One can still legitimately ask this question: how would such a system behave, even if we can’t solve it using this differential equation? If there were no friction and the bead were started off at the top with no motion, would it roll down? Alternatively, if you started it off with just the right speed to get to the top, would it stop there or continue to roll down the other side?
The answer is it would stop because when the body is at rest and there’s no external force, it will remain so. Newton’s laws of motion are more than one differential equation. If that equation does not give an unambiguous answer, that’s because it’s not the way to find the answer. That’s the difference between pure mathematics and physics.
In mathematics, you can make up any rules you want without regard to the real world. Physics is an empirical discipline. As Feynman noted:
It doesn’t matter how beautiful your theory is, it doesn’t matter how smart you are. If it doesn’t agree with experiment, it’s wrong.
In the present case, the experiment is nearly impossible to carry out because there are no frictionless wires or domes and there will always be perturbations that kick the mass off its unstable equilibrium. Nevertheless, Newtonian mechanics is well verified experimentally in other contexts. For well-behaved potential functions, the differential equation of motion has a unique solution for initial conditions of position and velocity. That is the Fundamental Theorem of ordinary differential equations.
Wilfred Kaplan, Advanced Calculus
